The calculation is intended for the purposes of geometric and strength designs of helical compression cylindrical springs made of wires and rods of circular sections, loaded with static or fatigue loading resp. In addition to the design of geometric and strength parameters, the calculation works with CAD systems. The application provides solutions of the following tasks:
Automatic design of a spring.
Selection of an optimal alternative of spring design in view of strength, geometry and weight.
Static and dynamic strength check.
Calculation of working forces of a spring of known production and installation dimensions.
Calculation of installation dimensions for known loading and production parameters of the spring.
The application includes a table of commonly used spring materials according to ISO, EN, ASTM/SAE, DIN, BS, JIS and others.
Support of 2D a 3D CAD systems.
The calculation is based on data, procedures, algorithms and data from specialized literature and standards EN 13906-1, DIN 2089-1, DIN 2095, DIN 2096.
User interface.
Download.
Purchase, Price list.
Information on the syntax and control of the calculation can be found in the document "Control, structure and syntax of calculations".
Information on the purpose, use and control of the paragraph "Information on the project" can be found in the document "Information on the project".
A compression spring is a helical cylindrical spring with constant spacing of active coils and approximately constant stiffness which is able to receive external forces acting against each other in its axis. In view of spring function, there are four basic states of springs:
| State of the spring | Description of states of a spring | index |
| free | the spring is not loaded | 0 |
| preloaded | the spring is exposed to minimum operational loading | 1 |
| fully loaded | the spring is exposed to maximum operational loading | 8 |
| limiting | the spring is compressed to full contact of coils | 9 |
The above-mentioned indexes are used in the calculation to specify individual parameters of the spring related to the given state of the spring.
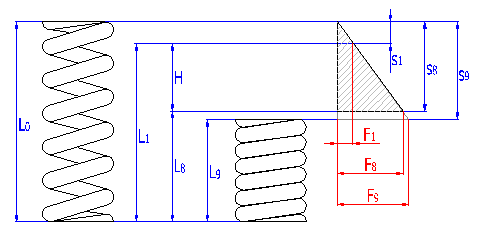
The task of spring design cannot be solved directly and allows considerable freedom in options of the design, dimensions or loading of the spring. Many springs of various designs and dimensions may meet requirements of the desired input parameters of the task. Therefore, it is necessary to proceed iteratively and successively evaluate individual designs of the spring. The calculation solves this problem by creation of a table of optimum designs following the chosen qualitative standard. The design procedure is given in the following items.
In this paragraph, enter basic input parameters characterizing the manner and mode of loading, design and method of seating the spring and parameters of the working environment.
Two basic methods of loading of springs are available for the purpose of calculation of springs:
Temperature of the working environment affects the spring relaxation, i.e. decrease in the force from the spring with its deformation to a constant length, depending on time. It is advisable to take this fact into account when designing the spring, and increase the level of safety during strength checks of the spring in case of temperatures over 80 °C. It is necessary to respect the working temperature also with selection of the spring material.
The service life of springs decreases significantly due to corrosion effects. Corrosion has very powerful effects particularly on springs exposed to fatigue loading. It is advisable to take this fact into account when designing the spring, and increase the level of safety during strength checks of the spring in case of a corrosion-aggressive environment. It is also necessary to consider corrosion effects with selection of the spring material.
In case of compression springs, it is always necessary to check its safety against side deflection. In addition to the size of the maximum working deformation (compression) of the spring, the manner of seating of the spring also considerably affects possible side deflection.
A spring which cannot be designed to prevent side deflection is usually supported using a centre pin or a sleeve. If there is a danger of damage to the spring due to friction with the pin or sleeve, the spring can be divided into several shorter springs arranged in series.
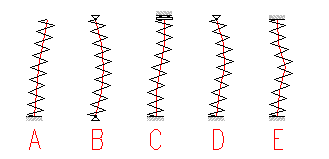
Select the type of seating in the list according to the illustration.
A) Fixed - free ends
B) Pinned - pinned ends
C) Clamped - clamped ends with lateral restraint
D) Clamped - pinned ends
E) Clamped - clamped ends without lateral restraint
F) Guided seating: the spring is guided inside a sleeve or on a pin
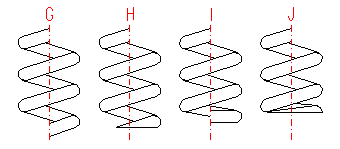
In case of compression springs, several various designs of spring ends are used. These differ in numbers of ends and machined coils and designs of supporting surfaces of the springs. Select the design in the list according to the illustration.
G) Open ends not ground: the edge coil is not bent to the next one, the supporting surface is unmachined
H) Open ends ground: the edge coil is not bent to the next one, the supporting surface is machined to a flat end perpendicular to the spring axis
I) Closed ends not ground: the edge coil is bent to the next one (it usually adjoins its free end), the supporting surface is unmachined
J) Closed ends ground: the edge coil is bent to the next one, the supporting surface of the spring is machined
Shot peening of the spring increases the fatigue limit in torsion by approx. 10 to 15%. In case of springs with shot peening exposed to fatigue loading, this allows users to reduce the consumption of material for production of the spring, reduce its dimensions and installation space, increase the working stroke or increase protection of the spring against fatigue breaks. Therefore, it is advisable to apply the technical requirement of shot peening to all springs exposed to oscillating loading. Due to technological reasons, only springs with diameters of wire over 1 mm are shot peened.
Right-hand winding of springs is preferred (dextrorsal helix); left-hand winding is used only if necessary due to technical reasons.
End coils
End coils are edge coils of the spring, co-axial with the active coils, whose angle pitch does not change during functional deformation of the spring. End coils create a supporting surface for the spring and with compression springs, one end coil is usually used at both ends of the spring.
Gound coils
Edge coils of the spring, machined to a flat surface perpendicular to the spring axis. Usually machined from three-fourths of half of the end coil up to its free end. Machined coils are commonly used only with springs with diameters of wires d > 1 mm.
Select the manner of loading which best meets the requirements of the entered
specifications.
Minimum permissible ratio between the permissible limit stress in torsion of the selected spring material and the actual maximum working stress t8in the spring coils. For a non-corrosive atmosphere and working temperature of the immediate vicinity of the spring up to 80 °C, and with regards to the course and mode of loading, it is advisable to choose a level of safety of compression springs in the interval from 1.05 to 1.3. Springs working at higher temperatures or in an aggressive environment should be designed with higher levels of safety.
With helical springs, the stress appearing in the spring coil at a given loading is calculated for simple torsion. Additional bending stress appears in the coil due to its rounding. Therefore, the stress is corrected in the calculation using a correction coefficient. As several different coefficients are commonly used, select in the list the correction coefficient which meets your local usage or recommendations of standards.
Select the loading mode which best meets the requirements of the entered
specifications.
Two fields of fatigue loading of springs can be distinguished with springs exposed to fatigue loading. In the first field, with limited service life of springs (lower than approx. 107 working cycles, the fatigue strength of the spring decreases with an increasing number of working cycles. In the field of unlimited service life (the desired service life of the spring is higher than 107 working cycles), the fatigue limit of the material and thus the strength of the spring remains approximately constant.
The level of safety gives the minimum permissible ratio between the fatigue strength in torsion of the spring and the actual maximum working stress t8in spring coils. For a non-corrosive atmosphere and working temperature of the immediate vicinity of the spring up to 80 °C, and with regards to the course and mode of loading, it is advisable to choose a level of safety of compression springs in the interval from 1.05 .. 1.25. When determining the level of safety, it is also necessary to consider suitability of the selected material for fatigue loading. With materials unsuitable for fatigue loading, it is advisable to increase the desired level of safety by up to 20%. Springs working at higher temperatures or in a corrosive environment should be designed with higher levels of safety. Particularly corrosion significantly decreases the service life of a spring exposed to fatigue loading.
With helical springs, the stress appearing in the spring coil at a given loading is calculated for simple torsion. Additional bending stress appears in the coil due to its rounding. Therefore, the stress is corrected in the calculation using a correction coefficient. As several different coefficients are commonly used, select in the list the correction coefficient which meets your local usage or recommendations of standards.
This paragraph can be used for selection of the spring material. Immediately after selection of material in the list, all information necessary for the design and calculation of the spring is displayed. If you need more detailed information on the selected material, or define or modify your own material, switch over to the material sheet "Material".
From the selection list choose the required processing of the spring. The cold winding shall be used for springs of ordinary sizes with a diameter of the wire up to 16 mm. Hot forming shall be used for the production of heavily loaded springs of greater sizes with a diameter of the over10 mm.
Select the spring material from the list. In addition to 5 user's materials, the list includes selected materials of one standard. If you wish to use materials from another standard, select the respective standard in the sheet "Material".
This paragraph includes information on recommended use of the selected material. The spring material should be designed with regards to the manner of loading of the spring and operational conditions. If you must use a less suitable material, this fact should be reflected in an increased level of safety in the design of the spring (see row [1.13] or [1.18] resp.).
Properties of the selected material described in rows [2.4, 2.6] are evaluated in five degrees (excellent, very good, good, poor, insufficient), the relative strength in row [2.5] in three degrees (high, medium, low).
This part gives all parameters necessary for calculation, independent of the diameter of the used wire.
This chapter includes strength characteristics of the selected material which are necessary for the design and calculation of the spring. The data characterizing strength of the material may be different for the same material depending on the diameter of the used wire. Therefore, the values given here depend on the diameter of the wire as given in row [4.8].
Maximum permissible stress of the spring material for infinite life and zero-to-maximum stress fluctuation.
This paragraph can be used for the design of the spring. The task of designing the spring often has many various suitable solutions for the given input conditions. The application, therefore, proceeds iteratively with the spring design and for the given input conditions, it passes through individual designs of the spring and a set of most advantageous solutions is selected following the chosen qualitative standard. The selected solutions are then offered in the form of a sorted table in which you can select a suitable design. The data on the selected spring are then displayed immediately in the chapter of results.
This part can be used for entering input data describing the basic parameters of the working cycle which have to be met by the designed spring. The first input column displays the desired value of the given parameter of the spring; the second column gives the permissible deviation from the desired value in the range 0-99%. If the designed spring has to meet the desired value of the given parameter, a zero deviation must be entered.
In this part it is necessary to specify various filters and marginal conditions of the design calculation. Their setting may significantly affect the course of the spring design and determine the speed, accuracy and quality of the design, the scope and number of suitable solutions and a qualitative standard for evaluation of the best designs.
If it is necessary to limit the outer diameter of the spring in its design (for example, if the spring has to be led in a sleeve), enable the check box at the beginning of the row and enter the maximum permissible value of the outer diameter of the spring in the input field.
If it is necessary to limit the inner diameter of the spring in its design (for example, if the spring has to be led on a pin), enable the check box at the beginning of the row and enter the minimum permissible value of the inner diameter of the spring in the input field.
Active coils of the spring are those coils whose pitch angle varies during functional deformation of the spring. When setting a fine division, the design calculation tests a higher number of different designs of the spring and is able to give a more accurate and higher quality solution. On the other hand, this naturally slows down the design calculation of the spring.
When designing the spring, it is not possible to proceed without certain dimensional limitations. Some dimensions or ratios of individual dimensions of the spring are limited by the recommended values specified by the respective standards and producers. This creates a file of marginal conditions which must be taken into account in the spring design.
Strictly following these marginal conditions may cause elimination of some advantageous solutions from the resulting design, which may exceed some of the specified limits, however, despite this, they may be acceptable. Due to this reason, it is possible to set a filter of the design calculation in this row. Such filter specifies the percentage of exceeding the limit dimensions of the spring. This brings more suitable solutions, however, on the other hand it is then necessary to visually check the selected solution in the chapter of results and consider acceptability of possible exceeding of the limit dimensions of the springs. Exceeding limit dimensions is indicated by a change in color of the parameter value to red in the chapter of results.
This row decides whether the spring will be checked regarding side deflection in the course of the design calculations. If the check is enabled, the resulting design eliminates all solutions which do not meet the requirement of stability of the spring shape. The manner of seating of the spring very considerably affects any possible side deflection (see row [1.6]). If the spring has to be installed with a guide, the check need not be performed.
If the check is not performed during the design, the calculations are faster and more suitable solutions are obtained. On the other hand, there is the need to perform the check by the user himself, by visual matching of the data in row [4.44]. If the spring design is unsuitable, it is necessary to select another designed solution or change the manner of spring seating. A spring which cannot be secured against side deflection is usually guided on a pin or inside a sleeve.
If this check is enabled, the resulting design eliminates all solutions which include a length of the fully loaded spring shorter than the minimum limit test length. If this check is disabled, it is advisable to perform a visual check of the designed solution by matching rows [4.24] and [4.30].
If this filter of solutions is set to "Yes", the resulting design eliminates all solutions with calculated levels of safety ss lower than the desired level of safety given in row [1.13]. In case of springs exposed to fatigue loading, this filter also eliminates solutions with calculated levels of safety sf lower than the desired level of safety given in row [1.18].
If the filter is disabled, the resulting design includes all solutions with calculated levels of safety higher or equal to 1. Due to the fact that the desired levels of safety are usually more or less accurate estimations and only rarely reflect the accurately determined value, whose exceeding could lead to damage to the spring, experienced users can disable this filter during execution of the design and consider the level of safety of the designed spring directly in the table of the design or in the chapter of results in row [4.42] or [4.49] resp.
This row sets the criterion of evaluation of the quality of individual suitable solutions of spring design. The best solutions are then offered to the user in the table. The standard of quality can be chosen from the list according to the following formula:
The design calculation of the spring works on the iteration principle. This row can be used for setting of the number of iterations in the calculation and it affects the speed, accuracy and quality of the design. Generally, the more iterations, the slower the calculation and the more accurate the solution. However, it is also advisable to take into account other aspects when setting this row.
The speed of the design is affected by the capacity of the computer and the type of design more than by the chosen number of iterations. At the same time, setting a high number of iterations need not always bring more accurate solutions for certain types designs. Generally speaking, it is usually sufficient to set a low or medium number of iterations for common designs. Use of a high number of iterations is more important for very free designs, where all or the majority of parameters of the working cycle in paragraph [3.1] entered with a considerable permissible deviation, and the desired diameter of the spring, is not limited by filters in rows [3.8, 3.9].
This part can be used to initiate the design calculation and then to choose a suitable spring in the table of designed solutions. With regards to the complexity of the spring design, it is not possible to perform the design calculation automatically always with a change to one of the input parameters, as can be done with other calculations on the sheet. The design calculation is initiated once when pressing the button in row [3.19]. Information on the progress of the calculation is displayed in the dialogue.
After completion of the calculation, a table of designed solutions is filled in and sorted and values of the best (chosen) solution are transferred automatically to the chapter of results. The table is sorted according to the criterion set in row [3.18]. The table of designed solutions can be re-sorted whenever using another sorting criterion.
If the design calculation was unsuccessful and no suitable solution was found, this fact is indicated by a warning message and the table of solutions remains in its original state. The following text gives some particular problems which may appear, and their possible remedies:
Meaning of parameters in the table:
| D | Mean spring diameter |
| De | Outer spring diameter |
| Di | Inner spring diameter |
| d | Wire diameter |
| n | Number of active coils |
| L0 | Free length of the spring |
| L1 | Length of the preloaded spring |
| L8 | Length of the fully loaded spring |
| F1 | Minimum working loading |
| F8 | Maximum working loading |
| t8 | Stress of the fully loaded spring |
| ss | Level of safety of a spring exposed to static loading |
| sf | Level of safety of a spring exposed to fatigue loading |
| m | Weight of the spring |
| quality | A comparative value showing the quality of the solution with regards to the chosen qualitative standard [3.15]. The lower the given value, the better the quality of the design. |
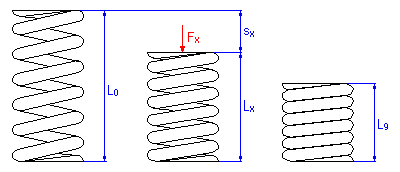
All necessary parameters describing the designed spring are shown in this paragraph for the given loading and dimensions of the spring. Entry data are transferred to the calculation from the table of solutions [3.20] of the chosen design of the spring or from some of the supplementary calculations [7,8,9]. For easier evaluation and check of values of individual parameters of the spring, some data are completed with their recommended limit values (shown in green fields in the listing). Exceeding of the recommended values is indicated by a change in color of the parameter to red. Critical values which might cause non-functionality or damage to the spring are indicated by a change in color of the whole field to red.
Parameters of the spring are divided in the listing into paragraphs according to the spring status; results of the performed strength checks of the spring are given at the end of the chapter. Meanings of individual dimensional parameters of the spring can be seen in the illustration.
If there is a need to tune some parameters of the designed spring (e.g. rounding of the designed dimensions), use some of the supplementary calculations [7,8,9] for this purpose

Pressing the button in this row refreshes values in the listing of parameters of the spring with the data from the design of the spring chosen in the table of solutions [3.20].
This parameter gives the ratio D/d between the mean diameter of the spring and the diameter of the used wire.
Theoretically determined limit value characterizing the maximum permissible deformation (squeezing) of a compression spring. This can be used to determine the minimum permissible test length of the spring [4.30].
If the spring is compressed to a length shorter than the limit length, the actual stiffness of the spring increases significantly above the theoretically determined stiffness valid in the field of compression to this length. At the same time, the critical speed decreases (see [4.34]) and this also increases the risk of mutual hitting of coils in operation. Due to these reasons, a compression spring (even during a test or installation) should not be squeezed to a smaller length. This results in the condition for the design of the spring that the length of the spring in a fully loaded condition [4.22] is greater than this limit length.
In case of compression springs with maximum speed of shifts of the moving end of the spring when loading or releasing is higher than their critical speed of squeezing, the inertia effects cause mutual hitting of coils. This leads to an increase in the actual stress in the spring coils by contact stress. This fact usually very adversely affects the service life of the spring and it is necessary to take it into account in designs of compression springs.
Resonance effects occur with compression springs exposed to fatigue loading. To eliminate these effects, it is necessary to load the spring at an excitation frequency different from the characteristic frequency of the spring (by approx. 15%).
The strength check of a compression spring is performed by comparison of the limit permissible stress in torsion of the chosen material [4.41] with the corrected stress of the spring in a fully loaded condition [4.40]. If the designed spring has to meet the strength check in the full extent, the resulting level of safety [4.42] must be higher or equal to the desired level of safety [1.13].
The stress in the spring coil is calculated for simple torsion and its calculated value is a theoretical value. In fact, the stress in the coil is higher because the curving of the coil causes an additional bending stress. Therefore, the stress is corrected using a corrective coefficient (see row [1.14]) for the purpose of a strength check.
In case of compression springs, it is always necessary to check its protection against side deflection. The check is performed by comparison of the maximum working deformation of the spring (expressed as a percentage of the free length of the spring) with the permitted deformation. The value of the permitted deformation is determined empirically for the given slenderness ratio of the spring L0/D and the type of seating of the spring. Generally, the risk of possible side deflection increases with an increasing value of the slenderness ratio and increasing value of the working compression of the spring. The manner of seating of the spring (see row [1.6]) has a significant effect on its possible side deflection.
A spring which cannot be designed as secured against side deflection is usually installed on a pin or inside a sleeve. If there is a danger of damage of the spring due to friction, the spring can be divided into several shorter springs arranged in series.
The strength check of a spring exposed to fatigue loading is performed by comparison of the maximum fatigue strength of the material determined for the given course of loading [4.48] with the corrected stress of the spring in a fully loaded state [4.47]. If the designed spring has to meet the strength check in the full extent, the resulting level of safety [4.49] must be higher or equal to the desired level of safety [1.18]. Naturally, even with a spring exposed to fatigue loading the condition of "static" strength check [4.38] must be met as well.
The stress in the spring coil is calculated for simple torsion and its calculated value is a theoretical value. In fact, the stress in the coil is higher because the curving of the coil causes an additional bending stress. Therefore, the stress is corrected using a corrective coefficient (see row [1.19]) for the purpose of a strength check.
Determination of the maximum fatigue strength of the spring is based on the ultimate fatigue strength of the chosen material and the given course of loading of the spring using a Goodman's fatigue diagram.
This paragraph can be used for calculation of parameters of a spring (designed in paragraph [4]) which is in a specific working condition. The paragraph [5.1] is designed for calculation of the length Lx of the spring, compressed by the given working force Fx. Paragraph [5.6] enables users to find the working force which is needed to squeeze the spring to the given length Lx.
This paragraph gives parameters of a strength check of a spring exposed to fatigue loading. The strength check of a spring exposed to fatigue loading is performed by comparison of the maximum fatigue strength of the material determined for the given course of loading [6.8] with the corrected stress of the spring in a fully loaded state [6.3]. If the designed spring has to meet the strength check in the full extent, the resulting level of safety [6.9] must be higher or equal to the desired level of safety [1.18].
The stress in the spring coil is calculated for simple torsion and its calculated value is a theoretical value. In fact, the stress in the coil is higher because the curving of the coil causes an additional bending stress. Therefore, the stress is corrected using a corrective coefficient (see row [1.19]) for the purpose of a strength check.
Maximum permissible stress of the spring material for infinite life and zero-to-maximum stress fluctuation.
Determination of the maximum fatigue strength of the spring is based on the ultimate fatigue strength of the chosen material and the given course of loading of the spring using a Goodman's fatigue diagram.
The first of the supplementary calculations can be found in this paragraph. This calculation includes three functions.
The calculation located in this paragraph includes two functions.
The calculation located in this paragraph includes two functions.
Information on options of 2D and 3D graphic outputs and information on cooperation with 2D and 3D CAD systems can be found in the document "Graphic output, CAD systems".
Information on setting of calculation parameters and setting of the language can be found in the document "Setting calculations, change the language".
When designing a spring, it is not possible to proceed without certain dimensional limitations. Some dimensions or ratios of individual dimensions of the spring are limited by recommended values determined by the respective standards (see e.g. DIN 2095, DIN 2096) and various producers as well. This creates a file of marginal conditions which must be taken into account in the design of the spring.
Therefore, different recommended limit dimensions of the spring may be used which can be modified in this paragraph according to the user's requirements. Minimum values of individual parameters can be entered in the first column, maximum values in the second column. In case of setting more free marginal conditions (by decreasing the minimum or increasing the maximum values), the application selects a suitable solution from a wider range of suitable solutions. This increases the chance of finding a solution of a better quality. On the other hand, this creates the risk that the chosen supplier will not be able to produce the designed spring.
If there are no special requirements for limit dimensions of the spring, the predefined setting can be used. Pressing the button in row [3.8] sets in input fields the implicit values corresponding to the file of marginal conditions for commonly delivered springs.
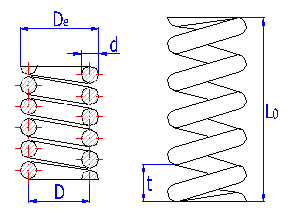
This gives the ratio D/d between the mean diameter of the spring and the diameter of the used wire. According to DIN:
4 to 20 - cold formed springs (DIN 2095)
3 to 12 - hot formed springs (DIN 2096)
Cold formed springs - according to DIN 2095, maximum 240 mm. There are commonly delivered springs with even greater diameters.
Hot formed springs - according to DIN 2096, maximum 460 mm.
It is not prescribed by the standard. Usually 1 to 10 with commonly produced springs. Increasing the ratio causes an increasing tendency to side deflection of the spring.
Cold formed springs - according to DIN 2095, maximum 630 mm.
Hot formed springs - according to DIN 2096, maximum 800 mm.
Springs of even greater lengths are commonly delivered.
Not prescribed by the standard, usual with commonly produced springs
0.3*D < t < 0.6*D - for wire diameters up to 10mm
1.5*d < t < 0.55*D - for thicker wires
Too small pitch of the spring usually prevents perfect shot peening of the spring.
A minimum of two active coils are prescribed for cold formed springs according to DIN 2095.
A minimum of three active coils are prescribed for hot formed springs according to DIN 2096.
General information on how to modify and extend calculation workbooks is mentioned in the document "Workbook (calculation) modifications".
With calculation of springs, it is not possible to intervene in the design calculation of the spring using modifications and changes in the workbook. With regards to the complexity of the task of designing a spring, this calculation is implemented as an internal function of the workbook.
^